Working in ${\rm I\kern -2.2pt K\hskip 1pt}(\underline{T})[\underline{X}]$.

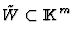The best result, when working in ${\rm I\kern -2.2pt K\hskip 1pt}(\underline{T})[\underline{X}]$, that we have found appears in [3] (pp. 277, Exercises 5 to 9), where it is proposed an algorithmic method to compute a subset $\tilde W\subset{\rm I\kern -2.2pt K\hskip 1pt}^m$ such that if $\tilde G=\{\tilde g_1(\underline{X}),\dots,\tilde g_s(\underline{X})\}\subset{\rm I\kern -2.2pt K\hskip 1pt}(\underline{T})[\underline{X}]$ is a Gröbner basis of the ideal $\tilde I=<\tilde F_1(\underline{X}),\dots,\tilde F_m(\underline{X})>\subset{\rm I\kern -2.2pt K\hskip 1pt}(\underline{T})[\underline{X}]$, and $t^{(0)}\in \tilde W$, then $\varphi^{(0)}(\tilde G)$ 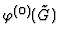is a Gröbner basis of 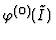$\varphi^{(0)}(\tilde I)$. This set $\tilde W$ of sufficient conditions on the parameters is defined by:

a)

none of the denominators of 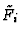$\tilde F_i$ are 0, $i=1,\dots,m$,

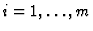b)

none of the denominators of $\tilde g_j$ are 0, $j=1,\dots,s$,

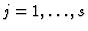c)

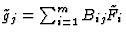let B_ij be the polynomials in ${\rm I\kern -2.2pt K\hskip 1pt}(\underline{T})[\underline{X}]$ such that $\tilde g_j=\sum_{i=1}^m B_{ij}\tilde F_i$; none of the denominators of B_ij are 0.

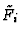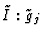Although the computation of the denominators appearing in the $\tilde F_i$'s and the $\tilde g_j$'s is easy, the computation of the denominators in the B_ij's can lead to lengthy calculations: we have to compute one quotient ideal ( $\tilde I:\tilde g_j$) and one elimination ideal ( $\tilde I:\tilde g_j\cap {\rm I\kern -2.2pt K\hskip 1pt}[\underline{T}]$) for every polynomial $\tilde g_j$ in the basis.