Kalkbrener's approach.

M. Kalkbrener (see [9]) proposes a slightly different approach, as he considers that the specialization map has the following domain and image:

$$\varphi:R\rightarrow {\rm I\kern -2.2pt K\hskip 1pt}$$

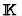where R is a Noetherian commutative ring ( ${\rm I\kern -2.2pt K\hskip 1pt}[\underline{T}]$ in our case) and ${\rm I\kern -2.2pt K\hskip 1pt}$ is a field, and a Gröbner basis G of I in $R[\underline{X}]$ with respect to any ordering in the monomials of $R[\underline{X}]$ (note that in this case Kalkbrener's approach requires the computation of a Gröbner Basis in ${\rm I\kern -2.2pt K\hskip 1pt}[\underline{T}][\underline{X}]$). The question to answer is: when is $\varphi(G)$ a Gröbner basis of $\varphi(I)$?, and the answer is similar to Gianni's one: in [9] it is shown that this is is equivalent to the following:

assume that the g_i's are ordered in such a way that there exists one $r\in\{0,\dots,s\}$ with $\varphi(lt(g_i))\neq 0$ for $i\in\{1,\dots,r\}$, and $\varphi(lt(g_i))= 0$ for $i\in\{r+1,\dots,s\}$. Then, for every $i\in\{r+1,\dots,s\}$ the polynomial $\varphi(g_i)$ is reducible to 0 modulo $\{\varphi(g_1),\dots,\varphi(g_r)\}$.

But remark that in order to test this condition, we need to compute a Gröbner basis of $\{\varphi(g_1),\dots,\varphi(g_r)\}$, but this can be, in the worst case, similar to compute a Gröbner basis of $\varphi(G)$, unless in the case in which r=s, where we can directly conclude that $\varphi(G)$ is a Gröbner basis. Although this approach seems to contain in particular Gianni's one, remark that G is a Gröbner basis in $R[\underline{X}]$ with $R={\rm I\kern -2.2pt K\hskip 1pt}[\underline{T}]$, which implies that it requires the computation of a Gröbner Basis over an integral domain ( ${\rm I\kern -2.2pt K\hskip 1pt}[\underline{T}]$) and not over a field which is the usual case: in [1] or in [2] it is described how to compute such Gröbner Bases.