Our investigation is dedicated to the construction
of doubly periodic classical fields in the (1+1)-dimensional
![]() theory. We study the model of an isolated real scalar field
theory. We study the model of an isolated real scalar field
![]() ,
described by the Lagrangian density:
,
described by the Lagrangian density:
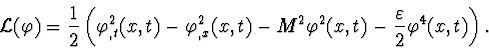
Let us consider the Lagrange-Euler equation:
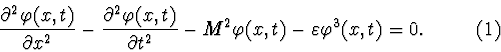
There are two classes of doubly periodic solutions for this equation. If we seek fields in the
traveling wave form:
The second class of doubly periodic solutions consists of functions in the
standing wave form:
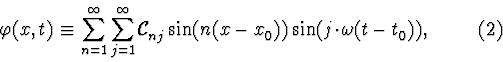
Exact standing wave solutions are not known. Approximate
solutions for equation (1) with
![]() and M=1 have been
found under the assumption that all highest harmonics are zeros:
and M=1 have been
found under the assumption that all highest harmonics are zeros:
![]() :
:
![]() ,
where N is a large
number. Numerical calculations have been made [5] for several
values of N. The obtained solutions are very close to each other, but
convergence of the sequence of these solutions as Ntends to infinity has yet to be proved.
,
where N is a large
number. Numerical calculations have been made [5] for several
values of N. The obtained solutions are very close to each other, but
convergence of the sequence of these solutions as Ntends to infinity has yet to be proved.
Provided that
![]() ,
C. Eugene Wayne [6] has considered
the problem of the construction of periodic and quasi-periodic in time
solutions for the equation (1) with the mass term, depending on
space-coordinate x. He has defined non-resonance conditions for
eigenvalues of the operator
,
C. Eugene Wayne [6] has considered
the problem of the construction of periodic and quasi-periodic in time
solutions for the equation (1) with the mass term, depending on
space-coordinate x. He has defined non-resonance conditions for
eigenvalues of the operator
![]() and proved that if these
conditions are satisfied, then one can construct periodic and
quasi-periodic solutions for equation (1), using a variant of the
Kolmogorov, Arnold, Moser (KAM) scheme. C. Eugene Wayne has remarked that
although his theorem gives one many periodic solutions, one has no
information whether or not they occur in smooth families.
and proved that if these
conditions are satisfied, then one can construct periodic and
quasi-periodic solutions for equation (1), using a variant of the
Kolmogorov, Arnold, Moser (KAM) scheme. C. Eugene Wayne has remarked that
although his theorem gives one many periodic solutions, one has no
information whether or not they occur in smooth families.